Reduced

Selling the following:
1) 1 Pair of SB Audience Rosso-65CDNT (1.41in) $195 ea New / Asking $160 Pair shipped SOLD

2) 1 Pair of SB Audience Rosso-65CD-T (1.4in) $170 New / Asking $140 Pair shipped SOLD

3) 1 Pair of SB Audience H280 horns $48 ea new/ Asking $35 shipped (must be with one pair of the above) SOLD

4) 3 QTY Satori MR16PNW-4 (midrange 4 ohm white cone). $190 ea New / Asking $260 shipped

All drivers are new, never mounted in cabinets. The SB Audience packaging is not mint, but will be well packaged. Satoris come in original packaging. Shipping CONUS only.

Reduced

Selling a pair of the Satori WO24P-4's. Purchased these recently but decided to go in a different direction. New-in-box. Never hooked up or mounted
$300 + CONUS shipping.

I've just received orders from the US sent to the UK. Both arrived in slightly under a week. Honestly, I couldn't believe it, and it got me thinking about all the moving parts that had to be in place for that to happen...

It's a thing that's always had something of a fascination for me ever since James Burkes series Connections. Saw it when I was (a lot) younger and it made me appreciate both the history of science and technology and the complexity of the webs of knowledge and experience that have to be in place for a thing to become an equivalent of an 'overnight' star.

The people, the computer systems, robots, trains, planes, automobiles and more than a couple of thousand years of blood sweat toil and tears combined to get me my stuff.

I really do feel genuinely honoured, lucky, privileged to be around now, to live in a world where such things are possible...

We are offering free PCB for JLH 2005 class-A amplifier
Please get it here:
Free JLH-2005 Class-A Amplifier PCB

Each order is limited to 2pcs.

If you don't have the components please go with this cheap kit:
JLH-2005 Class-A Amplifier Kit for only $24

I wasn't quite sure where to put this so if Design & Builds>Parts is more appropriate please Mod me to over there.

My little Mission M30i s have an HF down. I assume the ferro-fliud has dried out but the fabric dome and rubber suspension look worse for wear also. The other one is working but is outwardly as bad as the non-functional one. The XO is visually OK, no dry joints or dodgy looking caps.

They are Vifa units, LOGIC series OC25SC-04 which I believe are long since discontinued. While I have equiries in with Mission and sellers of NOS Vifa units, does anyone on here know a supplier that would probably have them? Ebay and Amazon are no goes as they don't function here.

If in europe I'd probably just buy new speakers but it's rather a HiFi desert here with only the two extremes available i.e. absolute rubbish or high end.

Thanks for your attention.

I've found some rather expensive 40410's and 40409's on ebay and I was wondering if someone knows of a NPN and PNP to replace these older RCA transistors.

This is a 'Thread Split' from here:
Simplicity and elegance, feedback wanted!

Selling the following:

Scanspeak Discovery 10F/8424G Pair: https://www.ebay.com/itm/275783153667

Lot of Various Small Drivers at Bargain Price: https://www.ebay.com/itm/275783171461

Please see my other speaker related sale listings.

Hi.
I have a Nad 317 that i changed most of the electrolytic capacitor , since the power board capacitors was way out of spec.
Amplifier works good besides one thing...it makes a pop in left channel when turning off.
I have separated pre amp with poweramp , to find out where the issue starts.
Issue is in the pre amp section , and the pop gets louder with increased volume.

This issue was there before i recapped the amp , and i also double checked tonecontrol board along with volumecontrol board and input board for cracked solderjoints.

Any ideas where i should look next for clues?

Ty

Hi people,

so my favorite headphones are broken. they were good open and light. Unfortunately they are not produced anymore.
I found them when i was looking for an alternative to coss porta pro but now i am searching again.

Please if you have some lightweight headphones recommendation for a reasonable price tell me.

ps: senheiser px 200 II are not an alternative. they are closed and hard to repair.

Hey folks.

Long time lurker of the great minds here. I purchased some inexpensive PA mids (PRV 10W650A-4) and wanted to fiddle around with a BIB style enclosure. Future plans aside, I just realized that the dust cap is mesh and the speaker has a vented pole piece. From my perspective, that sounds troublesome. I don't necessarily see that being a problem in a vented or BIB enclosure (maybe) but does anyone have experience with a speaker that has these characteristics and what to expect?

My first thought was a phase plug since but I also don't have measuring software and don't feel like paying for the shipping (Guam.)

Any inputs is appreciated.

Hey y'all, I purchased two of the ACA kits last year. Version 1.8.

I have completed the build of one but there is a cold solder on the right RCA input. A small wiggle and everything works fine.

The other kit is still in the box but has been opened.

If anybody is in the market for these wonderful sounding amps I am willing to let both of them go for the price of one.$349.00

The goal was to run them in mono configuration but have since moved on to other projects.

If interested, I can send pictures or additional information.

Thanks, David

Good morning all, I have a pair of ACA kits for sale. The 1.8 version

One amp has been completed and tested, working fine except for a cold solder joint on the right RCA input.

The other kit is still in the box but has been opened.

I am willing to let both kits go for the price of one $349

Hi all, I recently bought a set of spares or repair TDL Studio 3s. One has no drivers or tweeter. The other has a tweeter (labelled 25DT49 and marked 8ohm) and two 8" drivers (ELAC 8DC471). Note that these are the drivers with six bolts, rather than the four bolt square surround version.

From the Falcon Acoustics ELACIMFTDL spreadsheet (https://www.falconacoustics.co.uk/downloads/Elacimftdl.pdf), it appears that Studio 3s should have 16ohm 8" drivers, so it looks like I've got the drivers from a pair of Studio 2s in there.

I'm not sure all the wiring is original and I believe the crossovers may have been fiddled with. I've struggled to find other pictures of Studio 3 crossovers but these look a fair bit sparser in comparison. I'm no expert (couldn't be farther from it), but from studying the wiring on the pics I could find, I think the one tweeter is wired the wrong way around. Also it looks like the woofers are wired in series (positive from xover to speaker 1, negative from speaker 1 to positive of speaker 2, negative to xover) whereas I think the drivers should be wired in parallel?

Both of the woofer cones appear to be in good condition and move freely, but when I plugged the one speaker with drivers in it into an amp, only 1 of the drivers appears to make a little bit of noise, and I'm not sure the tweeter is working as it ought to be either.

I've done a bit of research and the only similar driver I can find is the Monacor SPH-8M (link: Monacor SPH-8M chassis speaker - Sound Services). I'm assuming I'll need four of these, and won't be able to re-use the 2 x 4 ohm ELAC drivers currently in the cabinets?

Any advice on these Monacors? Will they work? The T/S parameters don't look vastly different, but would appreciate any advice! I'm working on the assumption that I'd wire these 2 x 8 ohm speakers in parallel for a 4 ohm load?

Also, with regards to tweeters, there's not a great deal of info on the originals, but I only have one of them. I've been looking at the Audax 12x9 (link: Audax HD12x9D25 HD12x8D25 spares and replacement voice coils from Falcon Acoustics, The Leading Supplier of DIY Hifi Components.) but not sure if these would be suitable? Firstly, these would be 8ohm. If the 2 x 8ohm woofers would give me a nominal 4 ohm per cab, what impedance tweeter would I want? With the Audaxes above, it doesn't look like the holes on the 12x9 faceplates would be in the right place to attach to the speaker, so am I better off trying to get new tweeter faceplates fabricated and finding a different pair of tweeters to bolt to them?

On top of all this, I'm assuming I'll need to play about with the crossovers to get them working properly with the above speakers.

Any advice, suggestions or recommendations?

Cheers!

Inspired by tsardoz a few weeks ago, I've been making a lot of use of "tap" testing to identify the natural frequencies of my DML panels. It turns out to be quite informative. By "tap" testing, I mean simply striking the panel and measuring the response using the RTA feature of REW, with the microphone placed very close to the panel. This method is more correctly referred to as impulse excitation. Tsardoz called it "bash" testing! Curiously, I don't think I've ever seen anyone else share tap testing results on this or other forums concerning DMLs.

The most basic use of this technique is (as I mentioned above), simply to identify the natural frequencies of a panel. The frequency response of a DML is highly dependent on its natural frequencies. For example, the panels lowest natural frequency (i.e the fundamental), is approximately the lowest frequency at which can you can expect any significant output. Further, knowledge of the natural frequencies, along with the associated mode shapes (and hence the location of their nodes and antinodes), can help in exciter placement, since it can tell you where to place the exciter in order to excite (or not!) particular modes.

As an example, I will share some tap test results for a 14" by 48" plywood panel I've been working with lately, which was attached to a stiff frame using double sided foam tape around virtually the entire perimeter. In this case the lowest natural frequencies all look like the images below, which show the lowest three modes, that is, the fundamental (or 1,1) and the 1,2 and 1,3 modes, as well as one higher order (1.7) mode.




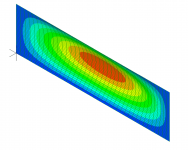
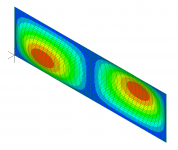
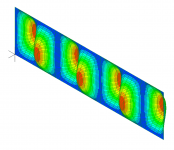
In these images, the red areas are the locations that exhibit the greatest displacement, and are called antinodes. When you tap a panel, the location where you tap determines which modes get excited (i.e. activated), and which do not. If you tap a red (antinode) location for a particular mode, that mode will be excited, while if you tap a blue location (node), that mode will not be excited. So, for example, tapping center of the panel will excite the 1,1 and 1,3 and 1,5 modes etc, since those modes all have an antinode (red) there at the center. On the other hand, tapping the center will not excite the 1,2 (or 1,4 and 1,6 modes etc) since it has a node at the center.
Likewise, the place where you position the microphone determines which modes you will detect. A mic will most easily detect the frequencies which have an antinode at or near the location of the mic.
For these reasons, finding the natural frequencies of a panel usually involves several measurements, made by tapping and mic-ing at a series of different locations.
In the case of my 14x48 panel attached to the frame around the perimeter, only two are needed to get most of the lower frequencies of interest. The first is tapping 2 or 3 inches from one one end of the panel, with the mic the same distance from the opposite end (and within about a 1/2" from the panel face). The other is simply tapping the center of the panel, with the mic also located at the center of the panel.
Here are the RTA measurements for the two tests:

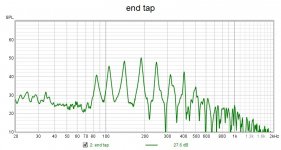
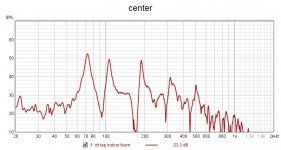
The first (end tap) shows a series of peaks which each correspond to a natural frequency of the panel. In fact, this captures all (or virtually all) of the natural frequencies of this panel below about 500 Hz, starting with the 1,2 mode at about 85 Hz, up to the 1,9 mode at about 500 Hz. Tapping close to the end, the fundamental was not detected in this first test. However, in the next test (center tap), the fundamental is detected at about 72 Hz. The center tap clearly excites fewer modes (half as many), since it only excites the odd,odd modes (1,1 1,3 1,5 1,7 etc).
Finally, it's interesting to overlay the tap test results with the REW frequency sweep frequency response measured at one meter from the panel. In this case, with a Dayton DAEX25fhe exciter. I placed the exciter about 13 inches from the center in the long direction of the panel, and about 1.375" from the center in the other direction. This was the location that provided the best (flattest) overall frequency response for this particular panel and mounting.


One thing I found particularly interesting is how the strongest peaks in the overall frequency response correspond exactly to the odd,odd natural frequencies that occur at 72 Hz (1,1), 107 Hz (1,3) and 188 Hz (1,5). And on the other hand, the overall frequency response shows no peaks at the odd,even natural frequencies such as 85 Hz (1,2) and 140 Hz (1,4).
We can learn a lot about how these panels actually work by doing testing of this sort.
Eric

I have a pair of orthodynamic headphones as in the title. They work fine despite of their age, but the soft foam parts that support on the rear side have deteriorated. They are made of foam, welded in a leather imitation of a special shape. See photo. Is there any chance to replace them? 3D printing perhaps?

First of all - what are suitable replacements for the defunct Fairchild J1943-O and J5200-O Transistors?
(There were also R-Types that seem to have distributed less power).
Russ was so kind to send me replacements 10 years ago, this weekend I had to use them.
The reason: The precise multi-turn trimmers were/are still too coarse to set the shunted current.
I accidentally turned the wrong ones. About two turns ramped the current up to 400mA which blew one trannie.
As it was R-Types I replaced all four.
And frankly, this board is not for repairs. Desoldering parts
of pushing a leg through a smaller hole makes the traces peel off.

But something must have changed during the swap of the trannies of the Placids.
I have two vintage CD-Players to compare, both running with ordinary 78xx/79xx regulators.
I also use a test CD (Sony YEDS-18) to play "digital silence" so that the relays do not mute.
Anyway, just by playing the silent track an ramping the volume of the amp
all the way up , the results are stunning:
- A vintage Sony CDP-X5000 shows hiss, which might be due to the 1-bit DAC
that has a lot of noise above the audio spectrum.

- A BuffaloII DAC driven by Placids, Single (I think 2.0.1) and BP (2.1.2) - reveals transformer/Mains hum!

- A vintage Philips CD-304MKII, driven with 78xx/79xx voltage regulators - is dead silent!
Even so silent you can hear the swing arm kicking, could be the servo signal bleeding into the audio circuit
or even be mechanical induction.

Interestingly something must have happened - in 2017 I wrote with the same test, the Placids were dead silent
and surpassing the Philips and Sony!

I have for sale this dac ifi zen dac v2 signature.
Very good sounding dac but i have to many right now.
Europe only - 220,00€ shipping registered included.
Original box, like new. used a few times.

Hi,

Never used or as new:

• 2* Beyma CP385ND 1p compression driver total 300€
• 2* Beyma SMC225ND 1p compression driver total 150€
• 2* RCF ND1411 1p compression driver total 90€

Good condition
2* Morel MW166 (can be used as Dynaudio 17W75 substitution) total 100€
2* DAVIS 13KLV5M 60€
1 CIARE 15.00SW 38 cm bass sub real linear 11mm xmax with good sensitivity 🙂 (Meyer sound used CIARE drivers in their famous subs) 150€

Scan Speak 23W-4557T02 revelator Top compact box sub driver 150€​

I am open to exchange against high quality tweeters ( scanspeak, biesma, sb acoustics, seas, morels AMT ... )
i can add money depending on what...

Pictures on demand
Available in France - Can ship worldwilde

Or the newer 8MC300Nd?

Interesting high efficiency midranges. Smaller than the 12p80Nd, yet larger than the usual 5 to 6.5" mids.

Hello,

slowing down the hobby to at least no new project or build. Here is what's left unused from various sources (including trades here!). Make offers and we will estimate shipping then, some parts as the Toslink kit may not be worth sending alone but can be a very cheap added to something else you want.


2x TPA Ventus boards
1x TPA Ivy I/V stage with OPA1632 and X7R supply decoupling caps (no signal filtering caps as it depends on your application)

An ESP project 113 board (from a trade but can't remember much...)



********************************************

Update:
Elma Switches sold,
UltraBiBs sold,
PFM Flea sold,
USB->SPDIF sold,
Toslink input sold,
R-Core TXs sold.
Ventus & IVY sold.
RPi sold.
********************************************

Thanks
Matthieu

This is an outgrowth of another thread. It was suggested that we needed a new thread where people could post their VituixCad simulations to enhance the general knowledge of cabinet diffraction issues and baffle layout.

It started with AllenB making a point:

Ironing a driver flat shouldn't be a problem if you choose the passband carefully, however there are many peaks and dips in measurements but many are not due to the drivers. If you EQ them all you may create problems.

Click to expand...

I added my thoughts:

To expand on what Allen is saying: It is very enlightening to experiment with VituixCad2 using ideal driver responses, and then simulate a 2-way speaker in a box.

Ideal drivers are the "default" option when you first open VituixCad, the drivers have a perfectly flat response. The next step is to use the diffraction tool and create some baffle shape, and then simulate the horizontal and vertical responses of the ideal tweeter and ideal woofer.

The next step is to build a crossover filter using the active elements available in VituixCad, such as low pass, high pass, notch, shelf, etc. Experimenting with active elements eliminates the complexities of impedance and electrical phase, and it allows us to concentrate on just the acoustical behavior of the simulation.

We might expect that with ideal, perfectly flat drivers, it would be very simple to get a flat response... however, if we simply slapped on a basic LR2, BW3, or LR4 crossover, we would not get a satisfactory result. We will often need quite a bit of filtering to achieve a good response. Baffle step is one obvious area we will have to deal with, but there are other diffraction effects as well. There are also acoustical interactions between the woofer and tweeter depending on their spacing, and on the crossover frequency and slope.

If the goal is a flat on-axis response and a good directivity index behavior, we might need quite a bit of experimenting in VituixCad to get a good simulation. What I found is that there are some baffle shapes/layouts which are easy to work with, and getting a good result is simple... There are other baffle shapes/layouts which are difficult to work with.

If there is an interest, I can post some of the more interesting results I have found... but I don't want to clog up this thread unless there is an interest.

Click to expand...

So that is what this thread is about. It would be cool if this thread becomes a repository for interesting simulations...

j.

Note: My first two sims in post #3 and #42 have an error, which is explained in the next dozen or so posts...

for fun i have tried to build a small 3-way, with 1” dome tweeter and 2” dome mid and 6” cone woofer, this far i am not sure it is any better then a straight 2-way using the same dome tweeter and 6” woofer, they sure sound different, by compersion the 2-way sounds edgy and bright and a bit nervous while the 3-way sounds polite and rounded and darker. The 2-way sounds more wild and fun and the 3-way sounds like an old person. Both speakers are built to measure similar both on and off axis.

what is your opinion on such speakers, a 2-way vs a small 3-way?

A few weeks ago I saw this tubetester for the first time on ebay:
DIGITAL TUBE TESTER - LAMPEMETRE AUTONOME ULTRA COMPACT | eBay

I wondered if it would be any good; it seemed to good to be true. I searched if there were people who had any experience with it, but could find nothing. There was some information, but it was all in French; not my strongest language🙄.

I decided to take the chance and order one, and put the experience here on diyaudio. Perhaps some tube lovers will benefit from it.

After I ordered it, I sent an email to the seller; "Rimlok" who had a very good reputation. I asked if he could sent me the manual in english in advance so I could start reading. I got an answer with the manual within 10 minutes, so that went well.

I ordered 20-1 and it arrived 24-1 so that was quick!

In about an hour I had it working and was testing my first tube; see picture.

The tester works really simple; it has two knobs for setting Va (anode/plate voltage) and -Vg (grid (bias) voltage).
If you push the test button it measures the Ia (anode/plate current). It measures pulsed so the tube is safe from overload.

You have to know what you're doing with these kind of testers, because you have to interpret the measurement yourself.
Manuals for vintage tubetesters are easily found on google; you can base setting on these, or use tube-curves to determine a measurement point.
For example: In an old AVO manual I saw that EL34 is mostly tested at Va 250v and -Vg -13.5v. According to the manual 75mA should be "new".

The meter measures in pseudo triode mode. There is an add-on in the manual to build a screen grid supply for true pentode measurements, but I decided to leave that be for now.

I quickly found out that al my old RFT EL34 are in different conditions, but not one broken. To my surprise, I had ordered 4 new electro harmonix el34 in october last year from "elektrodump.nl", 3 of the 4 tubes were defect. This was easy to see on the display, they were pulling grid current (gassy), so the negative Vg drops as the measurement starts. One of them even started flashing in the tube! As you can imagine I was a little bit disappointed... but after telling "elektrodump" my findings, they offered to replace the tubes free of charge! So the tubetester paid itself immediately back!

In the next posts, (if you are interested) the design/build experience, with some caveats here and there...🙂 (and a lot of pictures)

Paul

Taking on a new project in an area I know little about. Saw an ad for a fat tire ebike without battery for $200. Figured that was cheap enough that even if it was a mess, should still be worth it. Got it home and took a better look, and it is indeed a mess. Not only is the battery missing, but it's been hacked up pretty good. Everything inside the battery box is loose; in other words it's already a failed project. Looks like someone tried to swap in a non-stock motor controller already (looks like it might not even be for an ebike) with tons of unconnected wires, marrettes (!) and electrical tape. However, I still think I can make a go of it, it's a very simple bike, a Sondors fat tire ebike, just a single speed and a simple handlebar throttle controller, I think I can figure it out. I was hoping someone could point me to web resources that would help me. As in what is a GOOD ebike forum?

I’ve got a home build subwoofer using a Dayton SPA250 plate amp that I recently pulled out of storage. When I first powered it up I got loud thumping followed by a loud hum with the driver fully extended. (No signal hooked up). I opened it up and found some burnt resistors that I replaced. Now when I plug in I get a hum, very load when the switch is off, but quiets a bit when on. Again no input. If I send an input, it does play. The hum happens in my house and at my office so I think wiring is out. My next thought was the main filter capacitors. She I measure resistance across the caps, on one it starts low and gradually rises but on the other it sits at a constant 2500 ohm ( it’s in parallel with a 3k ohm resistor.) Should that be the next logical part to replace? Any help. Thanks!

Hello. I ask you to help me decide which of these three dacs is better to buy? As a priority, I would like to focus on the cheaper first option Rod Rain Audio DA68 ES9068AS, but I am confused by how the output circuit is oranized. Why are there only three operational amplifiers?

1. Rod Rain Audio DA68 ES9068AS

https://sl.aliexpress.ru/p?key=4PjSwv0

2. Es9038pro DAC

https://sl.aliexpress.ru/p?key=hCjSwRr

3. ES9038 ES9038PRO DAC 32bit DSD256 DAC Dual R-Core Power transformer

https://sl.aliexpress.ru/p?key=n6jSw9P

My snowbird neighbors finally showed up with the speakers they picked up for me in Indiana (I bought them off USAudiomart in Nov or early Dec) they just happened to live 30 min up the road but I would have to wait……………until they got here!
Tried them out and they were pretty much what I expected, smooth and clear even at high volume. Two things stuck out, one was an obvious difference in the top end between the two……seeing as these are from the 80’s I pretty much assumed the tweets and mids were gonna need rebuild and am packaging them up to send off to Richard So as he’s the man for ADS! (Actually the only man with parts)
The second thing was a bit more surprising, theres a quite noticeable box resonance in the upper bass / lower mids…..the bracing in the box only amounts to a 2” rib of 3/4” material on each side centered front to back and one piece same size across the front (see pic) I’ve never seen a box braced like this and its fairly obvious why not! So in answer i’ll just put in some cross brace 1” dowel in each direction…….Oh and I reckon there‘s a third thing that kindly surprised me is the orientation of the inductors all on the same plane!?

I’m going to recap the original xo‘s but I also want to build a pair from scratch with better quality components just for the heck of it (please no lectures on the waste of money, snake oil blah blah 😛) after tracing/mapping out the xo’s three times to make sure I got it right I end up with this (see pic) kindly odd can anyone explain that mid configuration? (See pic) tweet is reverse polarity and woofer looks normal.
Does anyone have a schematic for the L880/2 ? I’d like to figure out the inductor values without having to desolder them (unless the DATS V3 can measure accurately in circuit?)

This is the first ferrite core inductor i’ve ever had on a woofer(I take that back, my NS 670’s has them) can saturation come across sounding as a resonance?

edit; forgot to ask should I put a fuse in place of that polyswitch (if so what rating?) or just remove it?

edit II ; upon thinking about removing that polyswitch it dawned on me they do have a resistance even when closed….correct? if so would need a proper value resistor there, right?

Thanks,
MMB

Hello,

I am building an amp with 6SN7 driving interstage transformer as per schematic. I was told that it’s a bad idea to use cathode bypass capacitor on 6SN7 with an IT due to possible resonance. Removing capacitor is not really an option due to resulting impedance.



Would a fixed bias be a better option? For example a 6V battery instead of resistor and capacitor, would that work?

Thanks.

Hi!
I have a Denon PMA-1500RII with a blown channel.
It is equipped with 2SK851 N-channel mosfets.

Does anyone have a suggestion to a drop in replacement?
Can find a few on epay, but I don't trust it.

Cheers and salute, Jørgen.

It's classical schematic from the middle of 1980s. Scheme was took from here AIKIDO - Low-volage with buffer version
I don't know, who invented it, but using tube-preamp with solid state-out topology so strange, I guess... Anyway I decided to assemble this schematic, of cause without tube's preamp, for fun. 🙂
550C+560C may changed on 850C+860C NXP, ON Semi/Fairchild. In output I'm recommending to use MJE15034G+MJE15035G, or MJE243G+MJE253G instead crappy BD139+140 (doesn't matter ON Semi, NXP or NoName, cause their place in Power Supply only). PCB was made with standard "Star" topology and don't has any problems with hum, buzz or another crap.
DC offset doesn't exceed 30 mV (1st channel 1 mV float±2 mV, 2nd channel 25 mV and float ±3 mV). Bias 80 mA±2 mA (1st channel 78 mA, 2nd channel 82 mA and float ±2mA on two channels). One of the channels had problems on 50 kHz square signal caused by "broken" 1N4148. 🙁
The sound likes me a little more, than Lehmann BCL's Diamond Buffer (deeper sound scene, more details on the trebles and a little more aggressive bass).
p/s. It's only test assembling for fun. I like Diamond Buffer's schematics. It's a classic like Group B. I'm not recommending this scheme to amateurs, if you want DB, better start from Lehmann Black Cube Linear schematic or something passed test of time.

One channel is out
Does anyone know if these are fixable?
thank you for reading this

Got a kicker cxa1200, the 2045 was visually bad. Replaced it and still didn't power on. The diode d2 was out of tolerance so I replaced it and now it powers on but the drive doesn't look right. Did I cook the 2045 or is this how it's supposed to look without the power supply fets in?



Thanks

I am looking to upgrade a 4558 SIP OP AMP in a tuner
cant seem to find a better one at any reliable source, the ones I look at have no inventory of good audio SIP OP AMPs
anyone know where I can get a better SIP op amp that isnt fake?
I could get one on eBay but I have no confidence that they are genuine

Here is my long-time Philips friend that needs a nice, clean oscillator circuit. Please help my friend!


The popular, but closed Well Tempered thread is here:
https://www.diyaudio.com/community/...phase-noise-jitter-crystal-oscillator.261651/

I downloaded all of andrea_mori's PDF files but am a bit perplexed as to which particular circuit to use for my Philips, if any.
Maybe another circuit exists that's better suited to the old Philips osc.

Also, what ever happened to Laptech group buys? Can one still purchase them? Or osc's of equal quality from another manuf.?
Maybe the "cut" of the crystal isn't as important at the clcok/osc circuit it is encapsulated by? Yes?

Hi gang ... may I have the Sd for the newest Nirvana Super 8's (ferrite)?
Not listed and David is out.
Can't do MLTQWT without it.
Scored a pair of 12 x 72 hickory boards and really anxious to start on them.
Thanks, Zene

Can someone please tell me what to check in my Beringer mixer , the flash drive is not working

$35.00 OR BEST OFFER

1995 Audio Blue Book, Orion Research Corporation, 853 pages.
Contains very basic info on every piece of audio gear manufactured, Type, Years, WPC, Model Name, New List Price, Retail Used, Wholesale Mint & Ave.
Very little wear, almost as new, see images.
Pioneer takes up 23 pages, Marantz 12 pages.
Sold AS-IS I will be listing more classic audio and radio frequency engineering books, watch my listings.

$6.00 USPS Media Mail shipping to USA
$12.00 Priority/UPS shipping to USA. Will be packed with bubble wrap on all sides to immobilize in box and sufficient to prevent any damage from occuring during shipping.
Shipped promptly, tracking shared, safely packed.

To make purchase message me with your email address and I’ll send you an invoice from PayPal, Venmo or Cash App.

Hi everyone,

I have posted a blog about the performance of Wattgate 320i connectors vs. the Parts Connexion store brand. I hope you find it interesting and if you have constructive criticism please let me know.

https://inatinear.blogspot.com/2023/04/wattgate-320i-measurements.html

Hello, lately I was thinking about building V2 version of RH84 amplifier in closer unspecified future. Since ECC81 is not a cheap tube and I have E80CC in my possesion which has more gain than ECC82 but less than ECC81, I was wondering, if there is a possibility to adjust the schematic, for it to work with that E80CC. Has anyone done it? If not, what do I have to change, or rather how can I calculate the resistor in feedback circuit? Setting the right work point shouldn't be too hard, the only problem is with that feedback resistor.
Thanks

I have just finished designing a speaker that utilizes a p7hd full range driver and dayton sd270A-88 sub. The full range driver is in the open baffle area. I am not experienced with open baffle designs and would like to know if there are any are any glaring issues in that area. I am still working on bracing the exterior of the design so don’t worry about that aspect of the rigidity of this speaker. The blue outline around the front baffle represents a piece of acrylic which I am hoping will reduce the negative effect of edge diffraction. The acrylic sheet will be fused flush with the front baffle. The baffle size is 300mm wide by roughly 1000mm tall. I intend to create a passive crossover between the two and would also appreciate any advice on that end. Generally are there any big issues with this design.

Generally Specs:

Roughly 5 10” in height
Roughly 600mm wide at its widest point
Subwoofer box is separated from the full range driver.
Sub box: 400mm x 400mm x 250mm
Sub port: 3” port 225mm long (tunes to 35hz
Drivers:
Madisound p7hd
https://www.madisoundspeakerstore.c...pluvia-seven-hd-4-metal-cone-full-range-gold/
Dayton 10” sub
https://www.parts-express.com/Dayton-Audio-SD270A-88-10-DVC-Subwoofer-295-486?quantity=1
Designed in Fusion360

I have a Kenwood Model 500 i want to keep, an heirloom piece if you will, with this I want to gather a stock of spares for it. The 2SK58 I know you get from a source but was wondering if it was replaceable? I was thinking an LSK389b? What should I be looking at specs wise to match? What do you think?

Parts from a SPA 250 plate amp

$5+shipping for heatsink

$5+shipping for transformer

$5+shipping for case

$10+shipping for everything.

Recently I built some 15" coaxial surrounds (Vortex 15) for my home theater and I'm having an issue with what I believe to be standing waves in the box. The boxes are 48.5x17x12, ported and tuned to about 35Hz.

I ran an impedance sweep on the finished design and this is how it looks with no stuffing.


This is approximately what I'd expect. Resonance due to a standing wave of the longest dimension right around 140Hz. So I messed with putting a block of safe n sound insulation in the middle of the box, as well as trying a pillow in the middle of the box, and while they kill the resonance at 140Hz, a resonance shows up at 100Hz. See below.


It doesn't look that bad on the impedance sweep, but it is audible. Here's a nearfield sweep in REW. I plugged the port for this to make sure I wasn't getting any port weirdness.


I've built other box sizes with these same drivers/crossovers before, and didn't have issues, so I'm ruling out any issues with the drivers or crossovers. I built four of these new boxes and all of them exhibit the same behavior. All of them have drivers and crossovers pulled right out of the old boxes into these new ones.

I'm kind of at a loss of what to try next. I've done testing with the port plugged to make sure the port isn't the issue, and this issue is independent of the port. I've tried adding clamps and boards to the outside of the box to add more bracing to make sure it's not a panel resonance, and I haven't been able to eliminate it that way either. I'm hoping people on this forum will have some suggestions.

My game plan after work is to try putting a pillow right behind the driver, in addition to a pillow halfway down the box, and maybe some loose polyfill in the bottom of the box. But maybe you guys have some other ideas that could help.

Thanks for any and all help.

Hi,

A friend of mine has a Wadia 6 CD player for sale, but the Remote Control is missing.

Since this remote is almost impossible to find, does any of you knows the infrared codes emitted by the original Wadia remote ? if I get them, I could use a programmable universal remote !!

Thank you very much for your reply,

This thread is intended to be a place to discuss the balanced and unbalanced output versions of the stereo phono preamps as well as the Devinyliser designed by Doug Self and sold as boards only via The Signal Transfer Company website.
Website description: “The Signal Transfer Stereo Phono Preamplifier is a stand-alone version of the phono system used in the Precision Preamplifier. It offers both moving-magnet and moving-coil inputs, the latter with two gain options for optimal performance. It gives extremely accurate RIAA equalisation, a noise performance that approaches the theoretical limits, and superb linearity.”
No parts kits are currently available, according to recent correspondence with Mr Self.
Balanced output version: http://www.signaltransfer.freeuk.com/RIAAbal.htm
Unbalanced output version: http://www.signaltransfer.freeuk.com/RIAAunbal.htm
Devinyliser: http://www.signaltransfer.freeuk.com/devinyl.htm
Mr Self has taken over managing the website and fulfilling orders. He has been very responsive and the boards were received quickly.
I’m impressed with the build quality of the boards. Most of the parts are easily sourced using the BOM provided in printed documentation (or perhaps you will get a pdf) with the boards by searching for the values needed at your preferred supplier and verifying the part you choose has the correct lead spacing to match the board.
He does have a supply of the 2SB737 low noise transistors that he sells, tests each first and ships.
It took some digging and help from other diyaudio members to source a few of the parts.

Pending:
BOM with parts numbers from Digikey, Mouser, etc
Instructions to bypass Phono Preamplifier onboard subsonic filtering to leave that for Devinyliser to handle
Suggestions for some hard to source parts or their alternatives

Hi All.

Hoping you can advise me.

I need to replace the foam surrounds and cones on a pair of Jamo Speakers I picked up recently for $15.

I've removed the remains of what crumbled away and will soon be ready to glue their replacements.

What's left of the special glue (white) I bought won't be enough to finish the job.

Do I need to buy more dedicated foam surround glue or is standard PVA wood glue (white glue, elmers glue) suitable.


cheers

Cliff

Good day!

I am trying to amplify my audio recordings using the LM386 module and an ECM microphone. The module is connected to a laptop's USB port using a USB power cable. The audio plug is connected to a mobile phone for recording purposes. Whenever I try to record, only static noises are present. When I adjust the VOL in the module, loud crack noises are recorded. What am I doing wrong? Thank you.

I am hoping to build a small SE valve amplifier as a project. It is my first time building an amp and I am looking for some help. I posted another thread on here relating to a similar project salvaging parts from an (sadly broken) old tape recorder. However, it turns out I have different valves than I originally though and so, I have started a fresh with a new thread. I hope this is ok. I have chosen the schematic bellow as a starting point. I have a few questions relating to the components. Non of the resistors on the diagram a wattage rating. I'm not sure how to work this out in order to order suitable resistors.

For the HT 250v I'm planning to use a boost converter and I have an LM317 Buck converter for the heater's 6.3v supply.

I understand that in order to use the DC HT supple from the boost converter with the schematic bellow, some more work be required before the 1k resistors to remove ripple.

I'm using this build which uses boost and buck converters in place of a proper mains transformer as reference Login to view embedded media
The video above it described a build which uses a boost converter for HT supply. However, he uses a different tube and so his schematic is different to the one bellow.

In his build, after the boost converter before R3 and R7 he uses 2 x 100uf 400v caps and a 390 3W resistor (for smoothing?). I am unsure whether I would need to change the values here to suit the rest of my ECL86 schematic?

I'd be really greatful for any help figuring out how to adapt this to make it work with the schematic I've posted.


Kind regards!

Just want to bring attention to a neat PC based DSP recently posted in the PC forum.

https://www.diyaudio.com/community/...sp-for-crossovers-and-eq.397971/#post-7319282

I saw this on another audio forum. I'm actually not terribly surprised, perhaps only at the degree:

https://globalnews.ca/news/9589733/alan-cross-too-much-music/

A few years ago, I'd noticed a relative explosion of music titles, composers, and genres starting in the early 20th century in my own collection. This has now been taken a bit farther...by a few orders of magnitude.

Chris

Got this nice non-working Sherwood HP-2000. Got the preamp side working quite nice. The power section is a total wreck. One driver board is toast and the other one produces distortion and sets off the peak LED indicator. For now, I have taken out all the power section components and temporarily wired in circuit a class D Wondom power amp of the same rating (125 watts vs. 120 watts). Sounds wonderful!

But should I tackle rebuilding these driver boards and packing new outputs trannies (did one already)? I will need to match the input pair, pre drivers, and drivers to current transistors and think I will use the LME 48710 instead of the RCA3100 op amp.

I may just skip all this and go to a higher powered D class amp and call it a day.

I did calibrate the power meters with the new class D amp and got about 100 watt out of it with ease.

Some pics...I am sharing this thread as there is little info on these Sherwood nice vintage units. Although the driver board looks like it started a house fire, there is quite a bit of good left in it. I have highlighted in red the resistors that cooked off.

I talked with PapaZBill about this a long time ago and he suggested I start a thread. So here it is.

I've repaired this amplifier 3 times now. Every time it ends the same. Amp performs well. Amp dies at random. The first time is lasted a month. The second time, it lasted a week. The third time I decided to use it in my own vehicle to rule out 3rd party issues/abuse and subject it to an automotive environment. Perhaps witness it myself. It beat the brakes off my LMS 15's for nearly a month. Then one day, The moment it powered on - the PS popped. The track hadn't even loaded yet so it was signal free. The failure is always with one of the N channel devices. Never the same one really. I have used 3 different parts here. The 640, the 640n and 31n20d. I don't believe they're the cause of the problem. I've subjected this amplifier to high stress situations, lots of measurements, and every twist imaginable. I have not been able to replicate this failure mode on my bench. I was hoping someone knew something I don't to make this repair a 4th - and final - time.

Board is pictured. Thanks in advance.

I'm currently running a 2sk79 with a mu output from a Pimm self bias ccs. Looking at some alternatives I think I can run a ccs loaded stage from as little as 40v biased with about 20v on the output but I would like to use a b1 on the output instead of a mu output. The ccs I am thinking of using has a 5v minimum so that should leave plenty of swing for a pre. I think I have three main questions.

How can I modify the b1 to work in this situation? Is the cascode ZenMod suggested the way to go? Are there any existing schematics I can refer to?

Is there a better choice for an output buffer than a modified B1?

Is there a better choice for the ccs on top of the 2sk79. The bias point for the 2sk79 should be around 6ma with about 1.2v on the "source?" and 20v on the "drain?". So at idle it will drop 20v and I would want at least 10 v peak of swing, with I guess more being better. I have used this ccs before and it may be familiar to some, but I don't want to give details as I'm not sure it is in public.

Greetings DIYers. Yes, the title is exactly what I intend to build, as silly as it might seem.

I want to build a moderate power guitar amplifier using solid state actives. Why obsolete and salvage parts? Why solid state and not tubes?

There's a few reasons:

• I already have a few full power tube amps. They sound great at face-melting volumes, but their weight and volume levels make them slightly less practical for apartment practice or an impromptu jam or demo. A low-power tube amp would also fill this roll decently well, but still be heavy, and also be expensive. Post-COVID supply chain hangovers and the current war in Eastern Europe have constrained tube supply and made them even more expensive than they already were.
• I have access to bunch of obsolete and salvage parts through my own collection of stuff, and membership at a hackerspace. At least some of my BoM will be cheap or free this way.
• Being constrained by what I can get for extremely cheap or free will make this somewhat of a challenge and force me to get creative with the circuit design, and might even be fun.
• And finally, these older and salvaged parts are probably not going to run as clean and linear as modern parts would. I see that as a potentially useful quality in a guitar amplifier. Exactly how and why the poor electrical performance of old tube circuits creates the je ne sais quoi sought by guitarists has been discussed extensively, and IMO, still hasn't been fully worked out. But in either case, if bad electrical behavior can be pressed into a musically useful role, that bad behavior is possibly desirable to design for.

Here's what I have on hand so far:
1 120V:24V 40VA power transformer from a furnace
1 120V:16V 15VA doorbell transformer.
1 40W 10" speaker left over from a long-dead practice amp.
1 reverb tank, left over from the same long-dead amp.
A few hundred different transistors, in varying quantities

I am willing to buy some parts, if for no other reason than I know I'll have to. I'm going to need various resistors and capacitors for filters, voltage dividers, setting operating points for the transistors, etc. I'm even willing to buy a few complementary transistors, as I saw almost no complementary pairs in the parts drawers. I may have to purchase some voltage regulators as well, depending on what power supply circuits I am able to come up with. Beyond that however, I really would prefer to limit myself to the transistors on hand for the design, because that's the essence of this game: How good of a sound can I make with this old stuff, even if I have to force it into behavior it was never designed for?

Design Outline​

Here's the outline of the design that's been forming in my head over the past week:

• Class AB push-pull output section based on a complementary pair using some of the higher power transistors selected from my hackerspace's parts drawers, running at high voltage, into the primary of the doorbell transformer, stepping the voltage down and the current up to drive the speaker. Yes, I know this is unnecessary, appropriate BJTs or MOSFETs should be able to push enough current to drive the speaker directly. However, I believe the interactions between the output devices, transformer, and speaker are an important part of the vibe of the old circuits that have caught my ear. Thus, I think it is worth at least trying to design the output stage to use a transformer.
• A preamp section using a number of the small signal and not-so-high power transistors from the parts drawers, running at medium and lower voltages. Exactly how many gain stages, I'm not sure yet. I would like to span from nice clean tones, through edge-of-breakup, to a moderate crunch. Very high gain distortion is a maybe; we'll see what sounds I'm able to get out of the parts on hand.
• A power supply section running the furnace transformer, with a voltage doubler or quadrupler to get up to the voltages needed to make use of the doorbell transformer as an output transformer. Other portions of the power supply section can offer lower voltages appropriate for the transistors in the preamp section.
• Maybe use the reverb tank for a reverb circuit.
• All of this into a single combo chassis to keep the amp readily portable.

Timeline / Steps​

Here's how I see this going. I welcome any input and discussion as the project moves along through these phases. If this order of design is wrongheaded, I also welcome input on what might work better.

1. Transistor Selection​

   I need to work out which transistors are going to appear in the output and preamp sections. This will determine the voltage and current requirements from the power supply. I am leaning towards using the few JFETs and MOSFETs in the pile. This is mainly because I understand those are more readily coerced into similar misbehavior as the high-mu triodes and power pentodes that came to define the voicing of many tube amp circuits. However if some of the BJTs, SCRs, UJTs, or other miscellaneous parts available in my hackerspace's parts drawers might also be useful, I am interested.
   

2. Power Supply Design​

   I need to design a power supply to meet voltage and current needs of the transistors selected for preamp and power amp roles in the previous phase. I don't have any super firm ideas here yet. I like the designs I've seen from Walt Jung, but I don't know if those can be realized at both the high voltage I want in the output section, and the obsolete parts I'm limiting myself to for this project. I suspect I may end up starting a separate thread in the Power Supply section of the forums for this bit if crossposting is not against the rules.
   

3. Power Amp Design​

   Next, I will need to come back to the Power amp and work out its design in greater detail than the current vague idea of "high-voltage push-pull complementary pair across a doorbell transformer, because lol". This is where I expect a lot of the discussion on exactly how and why tube circuits misbehave and which of those misbehaviors are musically useful and worth attempting to replicate.
   

4. Preamp Design​

   Moving on to the preamp section, I expect even more time will be spent on how to get the transistors in this section to misbehave in fun ways, and how that misbehavior can be leveraged towards producing the kinds of voicing and character I want out of the amp.
   

5. Testing and tweaking​

   There should be some testing and tweaking of each of the above bits as they get built, but I expect there's going to be a lot more once start I connecting them together. I should anticipate lots of time playing riffs into the amp, critical listening, staring at bizzare oscilloscope traces with a "WTF?" look on my face, and coming back here with my observations to get help with the Why and How behind those WTFs.
   

6. Build the cabinet/enclosure​

   Honestly, this one gets into woodworking and cabinetry, which feels out-of-scope for this forum. If I remember to take picutres, I'll be happy to share them, though.

That's all I have for now. I am still working on transcribing the photos of my hackerspace's parts drawers. I figured I'd get this initial post up so I could get input on the basic design outline and the intended design process/sequence. I will have parts lists in my next post. They will be long. I am tempted to put them into tables for easier readability.

I bought P/F's The Wall on it's release day in November 79 in Brighton. I didn't get to play it at the time because my system was back in Rotterdam where I was living and working. I left it at my parents, so I first played it in 82. Some great tracks and it did make sense because I could identify with the trip the character was going through at that time in 79.

A few nights ago I saw that the film - The Wall was going to be on a TV channel here in France. Why I didn't get to see it on it's release in 82 I don't know. Watching the film brought it all back to me. Living somewhere I couldn't identify with - I don't like big cities, The rage and trashing of his apartment I understood completely why, a woman. I survived it all and came out stronger and not as a brick in the wall.

If you havn't seen The Wall - check it out.

I have built a few relay attenuators and I am very happy about the latest version.

However, one thing that I have not been 100% satisfied with is that there is no way of switching the relais during a zero crossing.

This can sometimes lead to glitches in the output, it is a common issue with all relay based attenuators and there is no easy fix for it.

Relais are just too slow and unpredictable for it to work.

I have thought about this problem for a long time and had a few ideas that I considered but nothing really seems feasible.

But then I got an idea.

What if you combine 2 relay attenuators and use an SPDT analog switch at the output of both.

The idea is that you start with the analog switch selecting Att. 1, this is your current volume setting.

You then change the volume of Att. 2, when that is done you wait for the zero crossing and the analog switch now selects the output of Att. 2.

Att. 2 is now selected and the volume level has now changed.

Now, if the analog switch is only allowed to switch between the 2 attenuators during a zero crossing, the final drawback with relay attenuators would be solved.

You continously switch between the 2 attenuators while you are changing volume, but only during zero crossings.

I have not done any schematic work yet, but I have a few ideas of how to implement it.

Am I totally crazy?

Can anyone identify the marking on a SOT-23-6?
N499t

This came out of a cut tape in a static bag that was supposed to be a stash of ZXTC2045E6TA's

Also does anyone know a good website with a comprehensive list of code markings?

LXmini Construction Plans V11. $75 OBO.

Hello dear diyaudio friends

For sale is a BPA300/PA150 LM3886 chip amplifier kit. It is a partial kit which includes the following parts:

4 x PA150 PCB, all SMD parts are already soldered (org. Shine7 PCB)
4 x partial part kits (kit do not include the four 2200uF 63V caps, connectors and input cap)
12 x LM3886TF (still in original National tube)
2 x fully assembled power supply PCBs
2 x empty power supply PCBs
16 x Panasonic 15000uf 35V capacitor (new)
2 x bridge rectifiers

Price for the complete amp kit: 150 Euro Sold


I’m also willing to trade for one or more of the following items:
(Of course, I will pay difference in value)
Manufacturer sealed bags of:

• 2SJ74-BL
• 2SK170-GR
• 2SJ74-GR
• 2SK370-BL

And maybe other rare signal JFETS or dual JFETS

• BurrBrown PCM1704-K DAC chips
• Analog Devices MAT02 and MAT03 dual monolithic transistors
• 2 x Starkrimson® Ultra Amp Module
• XRK Audio RTR TPA3255 Reference Class D amplifier

Please also take a look at my other offers in the Swap Meet

Shipping:

I will ship anywhere in the world

Everything together weights approximately 1,9KG, I'm not sure that I can fit everything into a package up to 2KG.
So the costs will strongly depend on your location. Shipping costs also strongly depend on the shipping method you prefer.
Of course, I will only charge the real costs, I won't charge anything for packing materials and effort. I prefer to use Deutsche Post/DHL, but you can decide which shipper and which shipping method I should use. Very secure packing by me is obvious.

As payment method I prefer PayPal for friends and family, so no extra fees will be added. Other payment methods on request. Of course personal collection of modules in Cologne (Germany) and payment in cash is also possible.

Kind Regards
Phil

I'm looking to build a small low gain or even unity buffer pre-amp to feed a low power class-A power amp (Sugden Musicmaster). I would like to keep it in the same footprint as the power amp, probably looking very similar to the current pre/headphone amp (Sugden Headmaster) which I hope to replace/improve on. This gives me a working area of about 200mm wide, 230mm deep and 50mm height to work within, hence I'm looking at miniature valves. I would like remote input switching (4 inputs, this can be simple switched relays) and remote volume (motorised Alps blue probably).

My skill level is more accurate copying than looking to come up with anything novel, hence I'm looking at SRPP designs and the Aikido and Aikido Cathode Follower designs. I can get away with very little gain, my current Headmaster has a gain of about 16.5dB which is more than I need (a 10dB pad would be handy). My main digital source has a 2V output which is enough to clip the power amp (1v input I believe) so a simple buffer with volume control and input switching could be viable. I have no intention of driving headphones from this, just the power amp.

I've looked at:
The Lampizator SRPP scribblings on his site: http://lampizator.eu/LAMPIZATOR/MIKRO/Mikrolampizator.html
The Aikido and ACF pages of the glassware site: https://glass-ware.stores.yahoo.net/newhardware.html
This Preamp: https://www.diyaudio.com/community/...wer-with-printed-circuit-board-design.206415/

Does anyone have any suggestions or experience they would be willing to share?

Many thanks.

On the FM3 schematic you can see two 1000µF capacitors, C113 and C114 :













But on the board there is one 1000 and one 680µF capacitors. My tuner has never been serviced, and I have seen the same configuration on others units.

Is there a reason for this ?
Should the capacitors be replaced as on the paper or as on the board ?

Out of curiosity bought some op-amps from aliexpress and the results were expected and unexpected. Current amp is the Douk U3 Mini(waiting for parts to build the sapphire4).
Only 2 functioning and 1 doa out of 25. The 3 that made it is Muses8920, LME49720, DEAD OPA1612 with adapter . The focus and the camera is not the best in my phone.


The stock Douk U3 came with a NE5532P, the audio was fine, but the massive pops at startup or when suddenly switching song was annoying so the curious me went to aliepxress and ordered the best in class op-amps.

NE5532P: Good sound, nothing to emphasize, maybe the top end was veiled a little bit and suffered when a lot of sound was playing at once.The DC at the output was 3V but it went down to 34mV after a 30 seconds. When turning it off, the offset jumped back to 3V to give me that nice pop. At startup the pop happened instantly then disappeared.

MUSES8920: Low end died a little bit and the top end felt more alive "aka" harsher and it became a guitar amp. The amp started to distort a lot when a loud sound happened, at first i thought it was my dac(cheapo usb c dongle) so thought nothing of it. After getting a cheapo es9018k2m dac the distortion remained so it wasn't the issue. The DC voltage at the output was 3.3V at startup and slowly went down to 50mV after 25min of idle. I could hear the "pop" slowly rise up the drivers then go away. 100% sure it was a fake MUSES chip.

LME49720NA: Low end returned with the high end becoming even harsher. I'm noticing more details in general and warmer tone, could be placebo or some "unwanted" harmonic distortion.
It doesn't distort at all and i need less volume knob turn to reach my listening volume compared to the NE and FAKE MUSES.
Oh boi the dc on this one. At startup its 18V then it dies down to 1mV in 20 seconds. I'm confident that this one is genuine. Not doing the pop sound test on this one.

OPA1612: Dead on arrival, nothing more to say about it.

In a 30min heat test. Case temp: NE 33°C, MUSES 31°C, LME 34°C, they all within 10%.

This "test" was done over 6 months.
Sidenote. HP is DT-990 250ohm. The amp is dead silent when nothing is playing and i don't have any issues others have reported with it. Its being powered by a phone charger 5V 2A.

Hi,

I need some experienced advise.
I'm restoring a NAD 2140 power amplifier and I've run into a problem that I don't understand, let alone find a fix.

The unit was blowing fuses and burning resistors with flames. Several transistors were out.
It is an early unit that had the extra stage before the output transistors, and has R639 1,2k and R641 180R.
I applied SB035 removing the -1 output stage.

I started by getting the regulator voltages in balance. Voltages were out of balance +45V and -41V.
This was done by tuning R813 to 24k (old version of amp has 22k, recent has 27kohm) and has now a clean +45V and -45.V, +25.9V and -25.7V.
The output transistors are fed with +51.1V and -51.1V, so within reasonable expectations.

I replaced all transistors, elco capacitors and resistances with quality items (Nichicon, Panasonic, Kemet, Vishay, ON, ...)

On powering up on DBT, all is OK, my 40W lamp goes to a very soft glow after the inrush current. Next was on 230VAC, no more fuses blowing, all resistors cool except R633 and R643, but within limits (I think).

The funny thing I don't understand is that all voltages towards the drivers are about 2V below what they should be. See attached scan with expected voltages in blue, actual voltages in red.
I have this issue on both channels, so clearly I made a mistake but after checking and double checking all R's, Q's and C's are according to the specs (except R639 1k2, R641 180R, old unit values).

Consequence is that I can't do anything that changes the idle current (VR603, I have 0 mV over the output emitter resistors), while I can change the output offset voltage to 0V (VR601).

Did anyone face this issue and how did you fix this?

A big thank you for your reply!
Chris

I'm building a speed controller that will have three adjustment pots for frequency and one for voltage. There seems no mechanism for preventing them spinning except friction with the inside of the face plate which is aluminum They are very free spinning but I don't want problems in the future. Suggestions?

Potentiometer

Hi, after playing with a dead Samson S700 amplifier with pretty good results after modification and upgrades, I've decided to also modify/upgrade my S500 tweeter amplifier.

The upgrade/modification was astonishing, the amplifier BIAS adjustment was very smooth and either pot adjust does not interfere with each other like previously did when adjusting, the previous hiss/noise was lowered significantly, however my tweeters now sound a lot clearer but also a bit analytical, I guess is due the possibly reduction in distortion/noise, however this is by hear and purely subjective opinion.

Parts used:
Profusion UTC 2SA1837 / 2SC4793 as drivers.
Toshiba 2SA1943N / 2SC5200N as VAS.

Between I've previously said that I will left the stock 2SA1492 / 2SC3856 Sanken transistors but opted to completely upgrade the whole unit regardless, also the modification is pretty much the same as I did on the Samson S700, note: I've also replaced the 47-ohm BASE resistors(R131/132/231/232) on the drivers for a 100-ohms fuse-resistors plus also replaced the BIAS pots with Bourns 3266W-1-103LF for smoother adjustments.

Here are the images of the modification and upgrade:
Jumpers closeup for reference.

Applying new thermal paste.

The amplifier module finished next to the removed lateral fets with the rest of its circuit parts, and the upgraded transistors/resistors/pots.

Module in place ready for testing and BIAS adjustment, I've used 150W the bulb for initially startup as usual.

Regards

Greetings friends, I'm back with this Angela Super Single-Ended project amp. If you're unfamiliar, here's the OG schematic.

Login to view embedded media
I built this with a few changes: only one rectifier (5Y3/5AR4), 12AX7 preamp tube, and a power transformer I had on hand, Hammond 276X, 640v@173mA. Unfortunately this PT is over-rated for this circuit, as I'm only pulling about 95mA with a pair of 6V6, the b+ is over 350v and thus too high for 6V6 tubes. Tinkering a bit more led me to try a pair of 5881 tubes for output, and while a 5Y3 rectifier runs 5881 tubes at about 75% of full bias (according to RobRob calc) a 5AR4 rectifier gives perfect voltages to bias the 5881s at 95% per RobRob. Schematic with voltages:

Login to view embedded media
So here's my question: How many watts are those 5881's making? Right now I have a Hammond 125ESE OPT, it's rated at 15w 80mA. Each 5881 is idling at 67mA if I'm reading this thing right. Am I gonna melt something? Would I be better off with a bigger OPT, or a smaller PT?

thanks!

About atenuation resistor in the high pass cut-off of midrange and tweeter for the caps and coils values calculation ?

I have a doubt, and need confirmation, please.

About passive filters please,

when choosing a cut off frequency, we need the impedance of the driver at this frequency to find for instance the value of the caps for the high pass.

1) If one is needing some attenuation by a resistor before the high pass, should he add the Z value of this resistor to the driver impedance at the cut-off frequency to calcul the caps value an coil one (if going second order or more) ?

2) What about the low pass of a mid as well when there is a serie attenuation resistor in the filter (or L-pad), please ? Should one add the attenuation resistor Z value to the DCR of the coil to have an idea of the magnitude behavior of the midrange driver in all its pass band (so from the high pass to the low pass in the order the AC signal sees it from the amp ? Rephrased differently : if the coil DCR is changing in the pass band used between the low end of the mid frequency (high pass of the cap) and the high end (low pass of the coil) of the midrange, does the total spl attenuation is varying according the frequency ( because of the non linearity in impedance of the coil) ?

I am also asking because sometimes we need to work with two drivers that have an important impedance gap where they met (cut-off frequency).

I do not know if the question makes sense but thanks for your answers.

Hello everyone,

I'm looking to re-cable my STAX earspeakers.
I'm looking to get the STAX Pro Bias 5 Pin Male Plug either from Allied electronics/Moon Audio.
However, I been told that it would be better to machine a plug out of Delrin with the gold connectors salvaged from a Neutrik 5 Pin XLR connector.

Which would be a wiser choice?
I'm leaning to the DIY as I can have more artistic control for the outlook of the plug
If I go for the DIY route using Delrin and salvaging those gold pins, anyone knows where I can get the CAD data for the coordinates for the Pro Bias?

Thanks you and have a nice day!

Hi,

https://www.diyaudio.com/community/attachments/ground-scheme-3-antiparallel-diodes-jpg.336247/

Anybody can tell me the values of the resistor and capacitor?

Thanks!

This thread was partly created in response to my query in another "I2S reclock" thread. There are several "I2S reclock" threads here on DIYA, a few 100s of pages long.
For now, I just want the simplest I2S re-clocker possible.
Something similar to:
https://www.diyinhk.com/shop/audio-kits/165-fifo-reclock-jitter-eliminator-for-8-channel-dac.html
IIRC, further tweaking is largely cleaning up pwr rails and decoupling.

Because I put away some projects over a decade ago, I was curious about this veroboard contraption in my "DIYA stuff" drawers.



It is a "sandwich" of: Kwack Clock v7 (bottom), I2S reclocker (middle), and Napier /2 (top). All connected togeter somehow (!!) and ultimately used for TDA1541 projects.

The I2S in the photo is based on three 74AC74 logic chips. One for each of the three I2S lines.

If you want to get your feet wet, bare-bones simple, just get three 74AC74 or 74HC74 chips. For starters, you don't even need the veroboard, decoupling caps /ferrites, or even 47R's. You can "fly" three chips with solid wire, like kites, to the pins of the(output I2S) decoder pins and (input I2S) DAC pins. And use any nearby +5vdc power.

That's it.

PS. My notes from the veroboard project say the orig design called for 74HC74. But for some reason, I wound up using 74AC74. Not sure why? Anyone know?

Hi,

I need to replace the 2SC4883 in a Marantz PM66KI. It's nothing to do with the output section, but used in a regulator that drives auxiliary circuits like the speaker relays, phono board, etc. The schematic is attached.

I feel like I should replace its friend the 2SA1859 too, as these transistors have been roasted for a couple of decades. Other cooked components in this part of the circuit already replaced. The 24V rail is sagging to 10V under load (7V when source direct relay is also engaged) and the transistor doesn't get warm anymore. I think it objected to me re-doing its fatigued solder joints as it was fine until I did that....

So I'm wondering what I can use to replace Sanken 2SC4883 / 2SA1859 with what is currently available.
The PM44 has the exact same circuit but uses 2SC3298 / 2SA1306 (Toshiba?) but these don't seem to be available either.

SANKEN 2SC4883 NPN 150V 2A 120MHz 20W TO220
SANKEN 2SA1859 PNP 150V 2A 60MHz 20W TO220

So what about:
ONSEMI MJF15030 NPN 150V 8A 30MHz 36W TO220
ONSEMI MJF15031 PNP 150V 8A 30MHz 36W TO220

ONSEMI MJE15030/1 are the same but 50W

I don't think the transition frequency of the transistor matters much in this application, but I am no expert.

Any advice welcomed.
Glenn

My clear intention is to get one from a thrift store/dumpster, even faulty, just for sourcing a Pacific Microsonics HDCD decoder.

Hi there,

Are there any circumstances under which it could be feasible to make a tapped horn based on a 12" subwoofer with only a 3" voice coil? It would likely be driven fairly hard as it's for live sound use. The only factor I can think of which might make it possible is the higher tuning, which would like to be about -3dB at 50-55hz.

thanks

hello everyone.
I am building a stereo speaker vented box. what are your thought on paring a dayton audio Ps-95 and a dayton audio AMT MINI-8 + a dayton audio 2 way 4500HZ crossover?

https://www.parts-express.com/OPEN-...ion-Transformer-Tweeter-86-275-095?quantity=1
+
https://www.parts-express.com/Dayto...Source-Full-Range-Driver-8-295-349?quantity=1
+
https://www.parts-express.com/Dayto...p=F&custcol_disableshopping=F&undefined=38.99

hello diyAudio members.

I am buliding a stereo speaker box using the PS-95 driver. before i start building I am using WINISD to simulate the box. my issue is as shown in the imeg, the impedance is off the chart when i set a 3.3L ported box and a 88 HZ resonant frequency. on the Dayton Audio and Parts express sites it is marked as a 3.3L 73hz F3 Vented box... what am i doing wrong?

I have a Kicker ZX750.1 that I have been running for years without issue until just recently. Lately, it would intermittently cut out. Yesterday I noticed it was not coming on at all so I decided to check it out. The red light will come on for a couple of seconds when I turn the system on and then the light goes out. The red light will also come on for a couple of seconds when the system is turned off. There is no light at all in between those times. I checked power, ground, and remote and they checked out ok. Nothing has been changed with the system. Any ideas on what would cause this or something else I can check? I would like to fix this myself if possible but I am fairly ignorant on the inner workings of an amp.

Idk what these are worth built 8 channels of the wire lpuhp and 100 was less then 64 so these are the extras.